Duality for abelian varieties

Let ${X}$ be an abelian variety over the algebraically closed field ${k}$ . In the previous post, we studied the Picard scheme ${\mathrm{Pic}_X}$ , or rather its connected component ${\mathrm{Pic}^0_X}$ at the identity. The main result was that ${\mathrm{Pic}^0_X}$ was itself an abelian variety (in particular, smooth) of the same dimension as ${X}$ , which parametrizes precisely the translation-invariant line bundles on ${X}$ .

We also saw how to construct isogenies between ${X}$ and ${\mathrm{Pic}^0_X}$ . Given an ample line bundle ${\mathcal{L}}$ on ${X}$ , the map

$\displaystyle X \rightarrow \mathrm{Pic}^0_X, \quad x \mapsto t_x^* \mathcal{L} \otimes \mathcal{L}^{-1}$

is an isogeny. Such maps were in fact the basic tool in proving the above result.

The goal of this post is to show that the contravariant functor

$\displaystyle X \mapsto \mathrm{Pic}^0_X$

from abelian varieties over ${k}$ to abelian varieties over ${k}$ , is a well-behaved duality theory. In particular, any abelian variety is canonically isomorphic to its bidual. (This explains why the double Picard functor on a general variety is the universal abelian variety generated by that variety, the so-called Albanese variety.) In fact, we won’t quite finish the proof in this post, but we will finish the most important step: the computation of the cohomology of the universal line bundle on $X \times \mathrm{Pic}^0_X$ .

Motivated by this, we set the notation:

Definition 11 We write ${\hat{X}}$ for ${\mathrm{Pic}^0_X}$ .

The main reference for this post is Mumford’s Abelian varieties.

10. The Poincaré line bundle and the biduality map

The first step in understanding the biduality of abelian varieties is to understand the universal line bundle on ${X \times \hat{X}}$ . By definition, ${\hat{X}}$ parametrizes line bundles on ${X}$ algebraically equivalent to zero, so there is a universal line bundle ${\mathcal{P}}$ , called the Poincaré line bundle, on ${X \times \hat{X}}$ .

The line bundle ${\mathcal{P}}$ has the property that ${\mathcal{P}}$ is trivialized on ${\left\{0\right\} \times \hat{X}}$ and ${{X} \times \left\{0\right\}}$ , and as ${y \in \hat{X}}$ varies, the various restrictions of ${\mathcal{P}}$ to ${X \times \left\{y\right\}}$ range exactly over the line bundles on ${X}$ algebraically equivalent to zero: this is precisely the definition of ${\hat{X}}$ .

As we’ve observed before, there is quite a bit of symmetry in this. Instead of regarding ${\mathcal{P}}$ as a family of line bundles on ${X}$ , parametrized by ${\hat{X}}$ , we can regard it as a family of line bundles on the dual ${\hat{X}}$ parametrized by ${X}$ . The result is that we get a canonical biduality map

$\displaystyle X \rightarrow \hat{\hat{X}}$

classifying the Poincaré bundle. This is a pointed map, hence a morphism of abelian varieties. The main goal of this post and the next is to show:

Theorem 12 For any abelian variety ${X}$ , the biduality map ${X \rightarrow \hat{\hat{X}}}$ is an isomorphism.

In particular, this implies a non-obvious fact about the Poincaré bundle ${\mathcal{P}}$ : as one restricts to the various fibers ${\left\{x\right\} \times \hat{X}}$ (for ${x \in X}$ ), one gets every translation-invariant line bundle on ${\hat{X}}$ exactly once.

The strategy in proving the above theorem is to show first that the biduality map is finite, by a diagram chase and the isogenies ${\phi_{\mathcal{L}}}$ constructed before. Next, we’ll show that the Poincaré bundle isn’t “redundant” on either factor by showing that its Euler characteristic is ${(-1)^{\dim X}}$ : this will imply that the biduality map is actually an isomorphism.

11. Finiteness of the biduality map

The first goal is to show that the biduality map ${X \rightarrow \hat{\hat{X}}}$ is a finite morphism: that is, it cannot annihilate a nontrivial abelian subvariety ${Y \subset X}$ . In other words, in terms of the Poincaré bundle, it states that for any ${Y \subset X}$ , the restricted bundle ${\mathcal{P}|_{Y \times \hat{X}}}$ is still nontrivial.

To see this, observe that ${\mathcal{P}|_{Y \times \hat{X}}}$ is a family of line bundles on ${Y}$ : it’s classified by the map

$\displaystyle \hat{X} \rightarrow \hat{Y}$

dual to the inclusion ${Y \hookrightarrow X}$ . The claim is that not only is this family nontrivial, but also that it hits every translation-invariant line bundle on ${Y}$ . That is, every translation-invariant line bundle on ${Y}$ extends to ${X}$ . In other words:

Proposition 13 If ${Y \rightarrow X}$ is a map of abelian varieties with finite kernel, then ${\hat{X} \rightarrow \hat{Y}}$ is surjective.

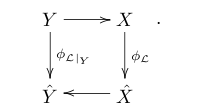
Proof: Given an ample line bundle ${\mathcal{L} \in \mathrm{Pic}(X)}$ , we have a morphism

$\displaystyle X \stackrel{\phi_{\mathcal{L}}}{\rightarrow} \hat{X},$

which classifies the bundle ${m^* \mathcal{L} \otimes (\mathcal{L}^{-1} \boxtimes \mathcal{L}^{-1}) \in \mathrm{Pic}(X \times X)}$ , for ${m: X \times X \rightarrow X}$ the multiplication.

The restriction ${\mathcal{L}|_Y}$ to ${Y}$ is still ample, since ${Y \rightarrow X}$ is finite, and we have a commutative diagram:

Since ${Y \rightarrow \hat{Y}}$ is surjective, it follows that ${\hat{X} \rightarrow \hat{Y}}$ is surjective. $\Box$

This in particular shows that the biduality map ${X \rightarrow \hat{\hat{X}}}$ must be finite.

12. The cohomology of ${\mathcal{P}}$

Our next goal is to compute the cohomology of the Poincaré bundle ${\mathcal{P}}$ on ${X \times \hat{X}}$ . (In particular, ${\mathcal{P}}$ itself is not algebraically equivalent to zero!) We can do this using the Leray spectral sequence and the categorified “orthogonality of characters,” although the argument is a bit technical, and we’ll split it into two sections.

Namely, ${\mathcal{P}}$ is a family of line bundles on ${X}$ , parametrized by ${\hat{X}}$ . By “orthogonality of characters,” each fiber ${\mathcal{P}_y, y \in \hat{X}}$ has vanishing cohomology except when ${y = 0}$ . It follows that the complex

$\displaystyle R p_{2*} \mathcal{P} ,$

which lives in the derived category of sheaves on ${\hat{X}}$ , is concentrated at the identity ${0 \in \hat{X}}$ .

If ${R = \mathcal{O}_{\hat{X}, 0}}$ is the local ring at ${0 \in \hat{X}}$ , we can localize this complex to get a complex ${P^\bullet}$ of ${R}$ -modules. The general yoga of base-change tells us that ${P^\bullet}$ is a perfect complex, and the (derived) tensor product ${P^\bullet \otimes_R k}$ is precisely the cohomology of ${\mathcal{P}}$ along the fiber: that is, ${H^\bullet(X, \mathcal{O}_X)}$ .

Let ${g = \dim X}$ . Since ${H^\bullet(X, \mathcal{O}_X)}$ is concentrated in dimensions ${[0, g]}$ , we conclude by Nakayama’s lemma that the cohomology of ${\mathcal{P}}$ itself is concentrated in dimensions ${[0, g]}$ . Moreover, the cohomology of ${\mathcal{P}}$ consists of artinian ${R}$ -modules, because it is supported at the origin.

That already buys us something. Roughly, there can’t be any cohomology close to zero, because then the derived tensor product with ${k}$ would blow that up into negative dimensions. (The grading is cohomological here.) In fact, we have a precise statement:

Lemma 14 If ${R}$ is a regular local ring of dimension ${g}$ , and ${P^\bullet}$ is a perfect complex of ${R}$ -modules with artinian cohomology such that the (derived) tensor product ${P^\bullet \otimes_R k}$ is cohomologically concentrated in dimensions ${\geq 0}$ , then ${P^\bullet}$ has cohomology concentrated in dimensions ${\geq g}$ .

Proof: Induction on ${g}$ . When ${g = 0}$ and ${R}$ is a field, it is evident. Assume that it is true for regular local rings of dimension ${g-1}$ .

We just have to prove that there is no cohomology below ${g}$ , by Nakayama’s lemma. Given a regular parameter ${x \in R}$ (in the maximal ideal), we can form ${P^\bullet \otimes R/(x)}$ , which is a perfect complex of ${R/(x)}$ -modules satisfying the same hypotheses. In particular, ${P^\bullet \otimes R/(x)}$ has no cohomology below dimension ${g-1}$ by the inductive hypothesis. Now consider the cofiber sequence

$\displaystyle P^\bullet \stackrel{x}{\rightarrow} P^\bullet \rightarrow P^\bullet \otimes R/(x)$

and the exact sequence in cohomology

$\displaystyle H^{i-1}(P^\bullet \otimes R/(x)) \rightarrow H^i(P^\bullet) \stackrel{x}{\rightarrow} H^i(P^\bullet).$

For ${i < g}$ , this means that multiplication by ${x}$ is injective on ${H^i(P^\bullet)}$ ; since these are artinian modules, they must vanish. $\Box$

13. The cohomology of ${\mathcal{P}}$ : part 2

Keep the notation of the previous section. Our conclusion is that the derived push-forward ${Rp_{2*} \mathcal{P}}$ must be supported at the point ${0 \in \hat{X}}$ , and concentrated in cohomological dimension ${g}$ . The claim is that the cohomology in dimension ${g}$ is precisely the ground field ${k}$ .

In fact, we start by noting:

$\displaystyle H^g( Rp_{2*} \mathcal{P}) \otimes_R k = H^g( X, \mathcal{O}_X) = k,$

by Serre duality (recall that ${\mathcal{O}_X = \omega_X}$ ). It follows that ${M = H^g( Rp_{2*} \mathcal{P})}$ has the property that ${M}$ is generated by one element. Moreover,

$\displaystyle \mathrm{Tor}^R_g(M, k) = H^0( Rp_{2*} \mathcal{P} \otimes_R k) = H^0(X, \mathcal{O}_X) = k.$

To see that ${M}$ is in fact ${k}$ , we will use a bit of local duality theory, as explained in this post. Namely, the statement is that the category of artinian modules over ${R}$ is dual to itself, via the local duality functor

$\displaystyle \mathbb{D}: N \mapsto \mathrm{Ext}^g(N, R).$

In fact, ${\mathbb{D}}$ is given by the derived maps from ${N}$ to ${R}$ , up to a cohomological shift.

In our case, the (artinian) module ${M}$ has the property that ${M}$ is generated by one element. That doesn’t mean that ${M= k}$ , though (not even that along with ${\mathrm{Tor}_g(M, k) =k}$ : take ${M = k[x]/x^2}$ over ${R = k[[x]]}$ ). We need to use an additional feature that can be seen using the local duality. The strategy is going to be to show that ${\mathbb{D} M}$ is isomorphic to ${k}$ , because ${\mathbb{D} M \otimes k \simeq k}$ and because the surjection ${\mathbb{D} M \rightarrow k}$ can’t be lifted further.

Let ${P^\bullet}$ be a finite complex of projectives quasi-isomorphic to ${M[-g]}$ (i.e. a “cofibrant replacement”). We observe that for any artinian ${R}$ -algebra ${S}$ , we have ${H^0(P^\bullet \otimes S) \simeq H^0( \mathcal{P}|_{X \times \mathrm{Spec} S})}$ , which cannot surject onto ${k \simeq H^0(\mathcal{P}|_{X \times \ast})}$ simply because ${\mathcal{P}}$ cannot be trivialized beyond ${X \times \ast}$ .

That last statement is a consequence of the fact that ${\hat{X}}$ is the solution to a moduli problem: if ${\mathrm{Spec} S \rightarrow \widehat{X}}$ pulled ${\mathcal{P}}$ back to a trivial bundle, then by definition it would have to be constant at the origin.

Now, we can write

$\displaystyle H^0(\mathcal{P}_{X \times S}) = H^0(P^\bullet \otimes S) = \hom( \mathbb{D}M, S),$

for ${\mathbb{D}}$ the local duality functor from artinian ${R}$ -modules to itself. In fact,

$\displaystyle H^0(P^\bullet \otimes S) = H^0( \mathbb{D} \mathbb{D} M[g] \otimes S) = H^0( \mathbf{RHom}( \mathbb{D} M, S)) = \hom(\mathbb{D}M, S).$

The conclusion on ${\mathbb{D}M}$ is that:

${\mathbb{D}M }$ maps to ${k}$ nontrivially. In fact, taking ${S = k}$ , we find that ${\hom( \mathbb{D}M, k)}$ is one-dimensional, so ${\mathbb{D}M \otimes k \simeq k}$ .
In particular, ${\mathbb{D}M \simeq R/\mathfrak{n}}$ for ${\mathfrak{n}}$ an ideal (containing some power of ${\mathfrak{m})}$ .
However, the map ${\mathbb{D}M \rightarrow k}$ cannot be lifted under the map ${S \rightarrow k}$ for ${S}$ any local artinian ${R}$ -algebra. This proves ${\mathfrak{n} = \mathfrak{m}}$ and ${\mathbb{D} M = k}$ .
Dualizing, we find that ${M = k}$ .

The conclusion that we get (from this calculation plus the degenerate Leray spectral sequence) is:

Theorem 15 The cohomology of the Poincaré bundle is given by:

$\displaystyle H^i(X \times \hat{X}, \mathcal{P}) = \begin{cases} 0 & \text{if } i \neq g \\ k & \text{if } i = g \end{cases}.$

Climbing Mount Bourbaki